Thermal advection
|
B. Geerts |
3/’02 |
Thermal (or heat) advection is the transport of sensible or latent heat by a moving fluid, such as air. Mathematically, heat advection equals
-U.· Ñ T
Where U is the wind vector, · the vector product, and Ñ T the vector temperature gradient. When the wind blows from a warmer to a colder region (i.e. a negative temperature gradient), there is warm advection, which is positive because it causes local warming. This explains the negative sign (-) in the equation. Three factors, then, make the heat advection larger (Fig 1):
- a stronger wind
- a larger temperature gradient
- a smaller angle between wind direction and temperature gradient, i.e. to maximize heat advection, the wind should blow normal to the isotherms
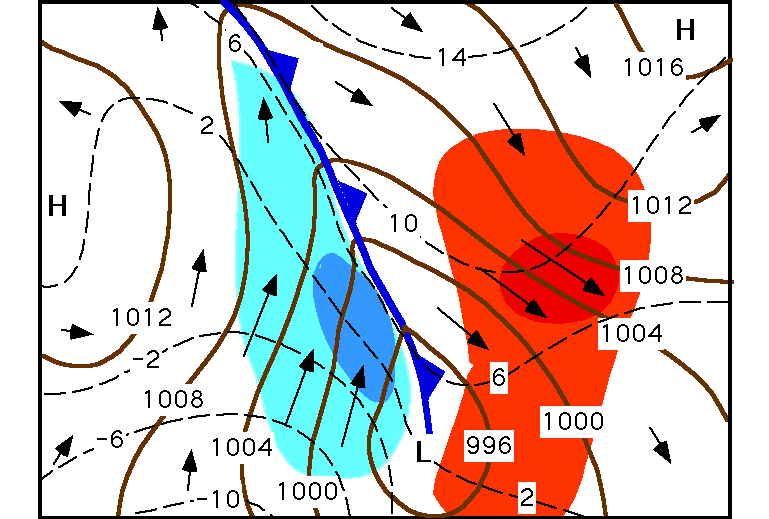
Fig 1. Heat advection. The thick solid lines are 1,000 hPa height contours (m). The geostrophic wind (which is shown by arrows) blows along these contours, and the real wind near the surface is close to the 1,000 hPa geostrophic wind. The length of the arrows is a measure of the wind speed. The dashed lines are 1,000 hPa isotherms (°C). Blue shading indicates cold advection, red shading is warm advection. A cold front extends from a low towards the north. The density of the shading is proportional to the amount of advection, i.e. the darkest area experiences the strongest advection.
Very little heat advection occurs at low latitudes on account of the very weak temperature gradients there. When the temperature is constant on a weather chart (to be specific, on a constant pressure surface, e.g. the 1000 hPa surface), the atmosphere is called barotropic. The low latitudes are generally in barotropic balance: there are no isotherms on the chart. Baroclinic conditions occur when a number of isotherms appear on a weather chart, so that (unless the isotherms are always parallel to the wind) thermal advection occurs. The special case of a chart where the wind blows parallel to the isotherms is called equivalent barotropic. On an upper level chart, this means that the isotherms and the height contours are parallel, because of near-geostrophic wind balance.
Thermal advection is a key factor in baroclinic instability (Note 13.B). Warm advection ahead of (to the east of) a frontal disturbance, and cold advection behind it, alter the thickness distribution and amplify the upper level short wave, thereby intensifying the frontal disturbance.
The sign of the heat advection (warm or cold) can be inferred from the wind profile. Such profile is measured by radiosondes but sometimes can be estimated by the movement of clouds at various levels. If in the southern hemisphere the wind veers (turns clockwise) with increasing height, there must be cold advection, according to the thermal wind balance (Note 12.F). Conversely, if the wind backs with height, warm advection occurs. In essence, this is because cold air is more compact than warm air (Note 12.E).
Frontogenesis is defined as the rate of change of the horizontal temperature gradient across a frontal boundary separating air masses of different temperature. A process is said to be frontogenetic if it increases the temperature gradient and frontolytic when it weakens the front. Horizontal advection is usually frontogenetic, because the airmasses tend to converge towards the front. In the case of Fig 1, horizontal advection clearly is frontogenetic. This convergence brings the isotherms closer together, i.e. frontogenesis occurs. Vertical motion near a front is usually frontolytic: the warm airmass rises over the front, thereby cooling adiabatically, and the cold airmass sinks, thereby warming. So the temperature gradient decreases.